Find the first three terms in the expansion of:
\((4a - 3b)^7\)
\(=16384a^7 - 86016a^6b \\+193536a^5b^2 ...\)
If £240 is invested with an interest rate of 3% compounded monthly, find the value of the investment after 8 years. £305.01
Here are the coordinates of 3 vertices of a square, what are the coordinates of the 4th?
\((1,1),(4,4),(-2,4)\)
(1,7)
\( X \sim N(65, 9^2)\)
Find
\( P(36\lt X \lt48) \)
\(0.0288\)
Factorise:
\(x^2-2x-8\)
\((x+2)(x-4)\)
Factorise:
\(4x^2-7x-2\)
\((4x+1)(x-2)\)
Draw a rough sketch of the graph of:
\(2y=x\)
Gradient 0.5
y intercept 0
What is the value of:
\(8^{\frac{1}{3}}\)
\(= 2\)
Find angle BCA if AB = 5.5m and BC = 7.2m. 49.8o
Find AC if angle ABC = 65o and BC = 3.6m. 3.26m
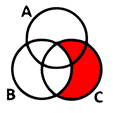
Describe the red region.
\(y = 3x^3 - 8x^2 + 6x\)
Find \( \dfrac{dy}{dx}\)
\(9x^2 - 16x + 6\)
\(y = \dfrac{8}{x^6} - 7\sqrt[8]{x}\)
Find \( \frac{dy}{dx}\)
\(-\frac{48}{x^7} - \frac{7}{8}x^{-\frac{7}{8}}\)
\(y=(5x^2-9)^5\)
Find \( \dfrac{dy}{dx}\)
\(50x(5x^2-9)^4\)
\(y=(3x+6)(8x-2)\)
Find \( \dfrac{dy}{dx}\)
\(48x+42\)
\(y=\frac{x+5}{x-2}\)
Find \( \dfrac{dy}{dx}\)
\(-\frac{7}{(x-2)^2}\)
Find the equation of the tangent to the curve:
\(y = 2x^2 - x + 3\)
where \(x = -1\)
\(y = 1 - 5x\)
Find the equation of the normal to the curve:
\(y = -4x^2 + 8x - 5\)
where \(x = -1\)
\(y = -\frac{x}{16} - \frac{273}{16}\)
\(y =21x^2 - 10x + 8\)
Find \( \int y \quad dx\)
\(7x^3 - 5x^2 + 8x+c\)
A game is played 11 times and the probability of winning is 0.9. Calculate the probability of winning exactly 10 times. 0.384
Make up a maths question using this:
\( A = 4\pi r^2 \)
Surface area of a sphere
What letter is this?
Two terms of an arithmetic sequence:
\(u_{7} = 30\)
\(u_{15} = 54\)
Find the sum of the first 48 terms.3960
Find the equations of the asymptotes of:
\(y=5\left(\dfrac{3x}{5+x}\right)\)
\(x=-5,y=15\)
In the triangle ABC,
BC = 8.2cm.
CA = 11.7cm.
BĈA = 41.7°
Find AB to 1 dp.
7.8cm
Evaluate:
$$\sum_{n=4}^{5} 109 - n^2$$
177
\(f(x)=-9x^2-6x-3\)
What is the value of the discriminent and what does it indicate?
-72, No real roots
\(f(x)=x^2+7x-8\)
By completing the square find the coordinates of the vertex.
(-3.5, -20.25)
Solve for x:
\( \log(x) + \log(29-x) = 2\)
\(x = 4 \text{ or } x = 25 \)
Find the integral:
\(\int 3xe^{x^2} \;dx\)
\(\frac{3}{2}e^{x^2}+c\)
Find the equation of the straight line that passes through:
(-1, 6) and (6, 20)
\(y=2x+8\)
Find the inverse of the function \(f\):
\(f(x)= \sqrt{x-9}\)
\(x²+9\)
\(f(x)=3x+2 \\ g(x)=2x^2 \\[1cm] \text{Find }gf(x)\)
\(18x^2+24x+8\)
Write in standard form:
\(a \times 10^2 \times b\times 10^3\)
where \(a \times b \) is a two digit number \((10 \le ab \lt 100)\)
\(\frac{ab}{10}\times10^6\)
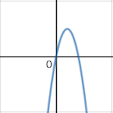
Draw a rough sketch of
\(y=x^3-4x\)
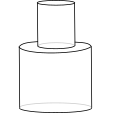
Sketch a height-time graph as this jar is filled.
Without a calculator find the exact value of
$$\cos{\frac{\pi}{4}} + \sin{45°}$$\(\sqrt{2}\)
Without a calculator find the exact value of
$$\tan{5\pi}$$\(0\)
Solve:
\(2d+3e-4f = 9 \\ d-e-f= -5\\ 9d+2e-2f=31\)
d = 3, e = 5, f = 3
Find the area of a sector with radius 8.3cm and angle \( \frac{\pi}{4}\)
🍕
27.1cm2
How many ways can twenty people be divided into two equal groups?
92378
Find the equations of the asymptotes of:
$$y=\dfrac{-6x^2+5x}{2x+1}$$x=-1/2,y=4-3x
The sum of the first 4 terms of a geometric sequence is 80 and the sum of the first 5 terms is 242. What is the first term?
2
Find the first 4 terms in the expansion of:
\(\dfrac{1}{(3+x)^2}\)
\(\frac{1}{9}-\frac{2x}{27}+\frac{x^2}{27}-\frac{4x^3}{243}\)
Evaluate:
\(\int^{\pi/3}_{\pi/6} \sin{x} \; dx\)
\(\dfrac{\sqrt{3}-1}{2}\)
29 Scouts went hiking. 15 got lost, 19 got blisters, and 10 got both lost and blisters. Find the probability that a randomly selected Scout got blisters, given that they were not lost.
\(\dfrac{9}{14}\)
Find the area of the triangle with sides:
\( \begin{pmatrix} 5 \\ 9 \\ 0 \end{pmatrix}, \; \begin{pmatrix} 9 \\ -9 \\ 3 \end{pmatrix} \; \text{and} \; \begin{pmatrix} 4 \\ -18 \\ 3 \end{pmatrix} \)
64.9 square units
Simplify
$$ (3+i)^{-2} $$
\(\frac{2}{25}-\frac{3}{50}i\)
Evaluate:
\(\int x^2 \ln{x}\; dx\)
\(\frac{x^3}{9}(3\ln x-1)+c\)
Simplify:
$$\cosec{x}\tan{x}$$\(\sec{x}\)
$$ \DeclareMathOperator{cosec}{cosec} $$Find the volume of revolution when \(y=\ln{x}\) is rotated about the y-axis for \(0 \le y \le 1\)
\(\approx 10.0\) cubic units
What is the difference between a permutation and a combination?
Permutations consider order; combinations do not.
Show how the first four terms of the Maclaurin series are obtained for
\(f(x) = \sqrt{x+1}\)
\(1 + \frac{x}{2} - \frac{x^2}{8} + \frac{x^3}{16}\)
Find the five 5th roots of 1
\(1, cis\frac{2\pi}{5}, cis\frac{4\pi}{5},\\ cis\frac{-2\pi}{5}, cis\frac{-4\pi}{5}\)
A committee of 4 is randomly selected from 8 men and 11 women. Determine the likelihood that it consists of all men.
35/1938 or 1.81%
Prove by mathematical induction that \( 5^n - 1 \) is divisible by 4 for all natural numbers \( n \)
Show true for n=1, assume true for n=k, prove for n=k+1
Write down a summary of your last Maths lesson focussing on what you learnt.
?
Tick (or untick) the boxes above to select the concepts you want to be included in this Starter [untick all]. The display at the top of this page will change instantly to show your choices. You can also drag the panels above so that the questions are ordered to meet your needs.
* Topics shown with an asterix are on the IB Higher Level syllabus but not included in the Standard Level syllabus.
This Starter is called Refreshing Revision because every time you refresh the page you get different revision questions.
Regularly use this Starter to keep that important learning from being forgotten. Here is the web address (URL) for the version of this page with your currently selected concepts:
Copy and paste the URL above into your lesson plan or scheme of work.
For more ideas on revision there are plenty of tips, suggestions and links on the Mathematics Revision page.
Answers appear here for Transum subscribers.
Try this Uniqueness Game with your class.
Transum.org/Maths/Game/Uniqueness/Game.asp?Level=8
Do you have any comments? It is always useful to receive feedback and helps make this free resource even more useful for those learning Mathematics anywhere in the world. Click here to enter your comments.
