

 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
Close

 | Click this icon to clear the pin board of all the lines you may have already drawn. |
 | This function allows you to undo the last line you drew. |
 | There are a number of settings you can adjust to change the way the pin board works. |
 | When you have constructed a polygon click on this tick to calculate its area. The calculation will give an incorrect answer if your shape is not a standard polygon. |
 | This is the home button. Click this to go to the home of Transum software. |
Close

You can change the size of the pin board:
Number of columns:
Shine + Write | Transum Mathematics | Topics
This is a visual aid designed to be projected onto a whiteboard for whole class exposition. It doesn't need to be an interactive whiteboard though of course that will make the resource more dynamic. The title "Shine+Write" suggests that the teacher or student can write on the whiteboard to enhance this visual aid.
The Transum Pin Board provides many ways to investigate two dimensional shapes. Clicking on the dots allows students or teachers to create shapes accurately, quickly and in a number of different colours. Use a screen clipping tool to capture the shapes you create for pasting into notes or alternatively copy the shapes onto square spotty paper.
How many different triangles can be constructed on the grid with 3 rows and 3 columns? How about 4 sided shapes? 5 sided?
Use the settings page  to select a grid of 20 columns. How many different quadrilaterals can be drawn on this grid? Change colour to begin a new quadrilateral.
to select a grid of 20 columns. How many different quadrilaterals can be drawn on this grid? Change colour to begin a new quadrilateral.
How many shapes can be constructed with an area of two square units?
How many different triangles can be constructed with just one pin inside?
Do you have any comments? It is always useful to receive feedback and helps make this free resource even more useful for those learning Mathematics anywhere in the world. Click here to enter your comments.
Here is the URL which will take them to a similar activity.
See the National Curriculum page for links to related online activities and resources.
Clive, Southampton
Wednesday, April 18, 2012
"Here's a challenge to 'Pick' at! Given a simple polygon constructed on the grid above, Pick's theorem provides a simple formula for calculating the area of this polygon in terms of the number I of lattice points in the interior located in the polygon and the number b of lattice points on the boundary placed on the polygon's perimeter. Can you figure out the formula?"
Will Emeny,
Thursday, September 8, 2016
"The following puzzle comes from the excellent Mr Barton's podcasts and was suggested by Will Emeny.
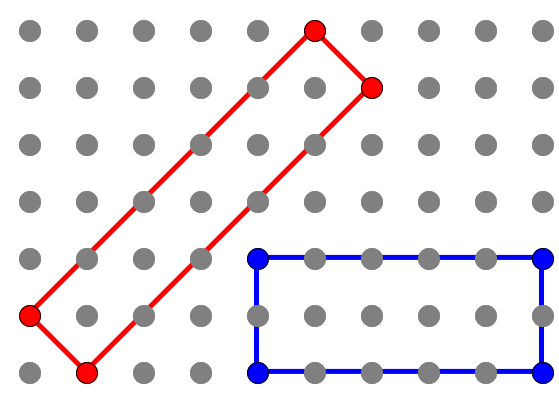
These two rectangles have an area of 10 square units.
In total, there are five different rectangles with vertices on grid points that have an area of 10 square units. Draw all five.
Prove there can be no more than five.
Draw all the rectangles that have an area of 12 square units. How do you know you've got them all?"