Recurring Decimals

Instructions Try your best to answer the questions above. Type your answers into the boxes provided leaving no spaces. As you work through the exercise regularly click the "check" button. If you have any wrong answers, do your best to do corrections but if there is anything you don't understand, please ask your teacher for help. When you have got all of the questions correct you may want to print out this page and paste it into your exercise book. If you keep your work in an ePortfolio you could take a screen shot of your answers and paste that into your Maths file.

	You are learning this because future ideas are built on previous ideas and the more ideas you learn the more power you have to learn anything, ever! The world's best football player engages in rigorous training, practicing skills repeatedly to prepare for the big game. While the specific actions rehearsed during these exercises are rarely needed in the match, the very process of performing these drills enhances their overall prowess as a top player. more...	More Activities: Maths Map Go Maths Games Puzzles Times Tables TablesMaster iPad Maths Investigations Exam Questions
Mathematicians are not the people who find Maths easy; they are the people who enjoy how mystifying, puzzling and hard it is. Are you a mathematician? Comment recorded on the 14 October 'Starter of the Day' page by Inger Kisby, Herts and Essex High School: "Just a quick note to say that we use a lot of your starters. It is lovely to have so many different ideas to start a lesson with. Thank you very much and keep up the good work." Comment recorded on the 12 July 'Starter of the Day' page by Miss J Key, Farlingaye High School, Suffolk: "Thanks very much for this one. We developed it into a whole lesson and I borrowed some hats from the drama department to add to the fun!"		Each month a newsletter is published containing details of the new additions to the Transum website and a new puzzle of the month. The newsletter is then duplicated as a podcast which is available on the major delivery networks. You can listen to the podcast while you are commuting, exercising or relaxing. Transum breaking news is available on Twitter @Transum and if that's not enough there is also a Transum Facebook page.
Featured Activity Nine Digits Arrange the given digits one to nine to make three numbers such that two of them add up to the third. This is a great puzzle for practicing standard pen and paper methods of three digit number addition and subtraction.	Answers There are answers to this exercise but they are available in this space to teachers, tutors and parents who have logged in to their Transum subscription on this computer. A Transum subscription unlocks the answers to the online exercises, quizzes and puzzles. It also provides the teacher with access to quality external links on each of the Transum Topic pages and the facility to add to the collection themselves. Subscribers can manage class lists, lesson plans and assessment data in the Class Admin application and have access to reports of the Transum Trophies earned by class members. If you would like to enjoy ad-free access to the thousands of Transum resources, receive our monthly newsletter, unlock the printable worksheets and see our Maths Lesson Finishers then sign up for a subscription now: Subscribe
Go Maths Learning and understanding Mathematics, at every level, requires learner engagement. Mathematics is not a spectator sport. Sometimes traditional teaching fails to actively involve students. One way to address the problem is through the use of interactive activities and this web site provides many of those. The Go Maths page is an alphabetical list of free activities designed for students in Secondary/High school. Maths Map Are you looking for something specific? An exercise to supplement the topic you are studying at school at the moment perhaps. Navigate using our Maths Map to find exercises, puzzles and Maths lesson starters grouped by topic.
Teachers
If you found this activity useful don't forget to record it in your scheme of work or learning management system. The short URL, ready to be copied and pasted, is as follows:	Alternatively, if you use Google Classroom, all you have to do is click on the green icon below in order to add this activity to one of your classes.	It may be worth remembering that if Transum.org should go offline for whatever reason, there is a mirror site at Transum.info that contains most of the resources that are available here on Transum.org. When planning to use technology in your lesson always have a plan B!
Transum, Wednesday, August 30, 2017 "Here's something to think about, discuss with your friends and share with your teacher: What is the difference between $0.\dot 9$ and one? " Ann Roberts, London Sunday, September 27, 2020 "Most fractions convert to recurring (repeating) decimals. Think of ⅓ converting to 0.333... There are some fractions that convert to terminating decimals. Think of ½ converting to 0.5. Which fractions convert to terminating decimals? I'll tell you. Write your fraction in its lowest terms n/d. Calculate the prime factorisation of the denominator d. Your fraction will only convert to a terminating decimal if: the prime factorisation of the denominator d contains ONLY 2s and/or 5s. In other words, the prime factorisation might include only 2s, or only 5s or a combinations of 2s and 5s. No other number is allowed. Let me show you. 1/20 converts to a terminating decimal because the prime factorisation of 20 is 2x2x5 (so only 2s and 5s). 1/9 converts to a recurring decimal because the prime factorisation of 9 is 3x3. (3s are not allowed). Use this rule to see if you can find some more fractions that convert to terminating decimals." Do you have any comments? It is always useful to receive feedback and helps make this free resource even more useful for those learning Mathematics anywhere in the world. Click here to enter your comments.

For Students:

For Teachers:

For All:

Help

A decimal with a repeating digit (or set of digits) is called a recurring decimal.

For example $0.77777777...$ is a recurring decimal and is called "nought point seven recurring"

$9.247347347...$ is also a recurring decimal and is called "nine point two four seven recurring"

The period of a recurring decimal is the number of digits in the repeating section so for the second example above the period is three.

A more efficient way of writing out a recurring decimal is by only writing the repeating digit once but putting a dot over the first and last number in the repeating sequence. Another method is drawing a line over the repeating digit or digits.Here are some examples.

$0.333333333... = 0.\dot 3 = 0.\overline 3$

$0.76531531531... = 0.76\dot 53\dot 1 = 0.76\overline{531}$

A fraction can be converted to a decimal using long division; dividing the numerator by the denominator. If the decimal is recurring the repeating pattern of numbers will be spotted in the long division working. The following example shows the repeating patterns when converting $ \frac{7}{11} $ to a decimal:

$Using long division to convert a fraction into a recurring decimal$

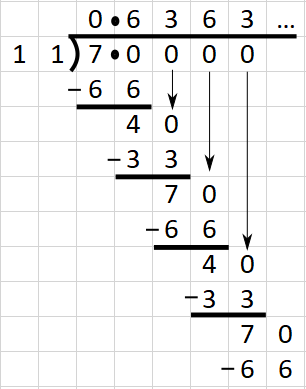
There are two common methods for converting a recurring decimal to a fraction:

Method 1

1 repeating digit

Let the recurring decimal be represented by $x$

$$x = 0.8888888...$$

Multiply both sides by 10 (as there is one repeating digit)

$$10x = 8.8888888...$$

Subtract the first equation from the second

$$9x = 8$$ $$x = \frac{8}{9}$$

2 repeating digits

Let the recurring decimal be represented by $x$

$$x = 1.36363636...$$

Multiply both sides by 100 (as there are two repeating digits)

$$100x = 136.36363636...$$

Subtract the first equation from the second

$$99x = 135$$ $$x = \frac{135}{99}$$ $$x = \frac{15}{11}$$

3 repeating digits

The method is the same but multiply both sides by 1000.

Method 2

1 repeating digit

Example: convert $0.8888888...$ to a fraction.

This method requires you to know that $\frac19 = 0.1111111...$

$0.8888888...$ is exactly eight times $0.1111111...$

$$\therefore 0.8888888... = \frac{8}{9}$$

2 repeating digits

Example: convert $0.45454545$ to a fraction in its lowest terms.

This method requires you to know that $\frac{1}{99} = 0.01010101...$

$0.45454545...$ is exactly forty five times $0.01010101...$

$$\therefore 0.45454545... = \frac{45}{99}$$ $$0.45454545... = \frac{5}{11}$$

3 repeating digits

Example: convert $0.\dot 61\dot 2$ to a fraction in its lowest terms.

This method requires you to know that $\frac{1}{999} = 0.\dot 00\dot 1$

$0.\dot 61\dot 2$ is exactly six hundred and twelve times $0.\dot 00\dot 1$

$$\therefore 0.\dot 61\dot 2 = \frac{612}{999}$$ $$0.\dot 61\dot 2 = \frac{68}{111}$$

Don't wait until you have finished the exercise before you click on the 'Check' button. Click it often as you work through the questions to see if you are answering them correctly. You can double-click the 'Check' button to make it float at the bottom of your screen.

Answers to this exercise are available lower down this page when you are logged in to your Transum account. If you don’t yet have a Transum subscription one can be very quickly set up if you are a teacher, tutor or parent.

1. This is a recurring decimal number: \(0.22222222\)... Which digit is recurring?
2. This is a recurring decimal number: \(0.43999999\)... Which digit is recurring?
3. A recurring decimal number can also be written like this: \(0.\dot 5\). Which digit is recurring?
4. For this number: \(0.17\dot 8\dot 2\). Which group of digits are recurring?
5. For this number: \( 0.198 \dot 834 \dot 0 \). What is the period of the recurrence?
6. Convert the fraction \( \frac{1}{3} \) to a decimal correct to 3 decimal places.
7. Convert the fraction \( \frac{2}{3} \) to a decimal correct to 5 decimal places.
8. Convert the fraction \( \frac{4}{9} \) to a decimal correct to 3 decimal places.
9. Convert the fraction \( \frac{10}{11} \) to a decimal correct to 5 decimal places.
10. Write the number \( 9 \frac{11}{12} \) as a decimal correct to 7 decimal places.
11. Write the number \( 9 \frac{8}{13} \) correct to 16 significant figures.
12. What is the period of recurrence of the decimal representing the fraction \( \frac{9}{13} \) ?

Recurring Decimals

Change recurring decimals into their corresponding fractions and vica versa.

Level 1 Level 2 Exam-Style Help More Decimals

Instructions

More Activities:

Featured Activity

Nine Digits

Answers

Go Maths

Maths Map

Teachers

Help

Method 1

1 repeating digit

2 repeating digits

3 repeating digits

Method 2

1 repeating digit

2 repeating digits

3 repeating digits