Sign In | Starter Of The Day | Tablesmaster | Fun Maths | Maths Map | Topics | More
This is the Transum Newsletter for the month of June 2023. It begins, as usual with the puzzle of the month.
x squared equals sixteen to the power of x. Find the value of x.
Let me know if you find a solution and I'll reveal my solution next month. While you think about that here are some of the key resources added to the Transum website during the last month.
The Brackets online exercise has been updated and although the questions remain the same the interactivity of the answer spaces has been updated to the common standard used for inputting mathematical expressions.
A new Level 9 has been added to the Indices exercises. Each question is a puzzle similar to, but easier than, the puzzle of the month above. A sound knowledge of the rules of indices is required.
Refreshing Revision, the Advanced version, now has some nice graph sketching questions as well as complex numbers questions for students taking the higher level courses. I designed them with a particular student who is taking the International Baccalaureate Analysis and Approaches Higher course but I hope they are useful for your A-Level students too.
A new Conditional Probability exercise has been created. There is only one level so far but I'm thinking that may be enough. Let me know if you think I'm missing any twists on those 'given that' situations.
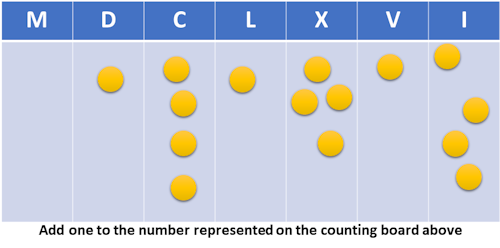
I'm contemplating the creation of an interactive web page dedicated to a Medieval Counting Board, also known as a Roman Numeral Abacus. This idea was inspired by an exhibit at the superb Almonry Museum in Evesham, which I had the pleasure of visiting last month. The concept of a 'reckoning board' was novel to me, but I immediately envisioned it as an intriguing challenge for upper Primary students.
My initial thoughts are to have the columns representing all of the Roman numeral symbols rather than just the powers of ten in order to develop a deeper understanding of place value from a number system that famously does not have place value.
In the last few newsletters I have been sharing my experiences of using artificial intelligence and ChatGPT in particular. Here is my latest question:
Me: "In your experience of reading web pages and social media exchanges what are the five most common misconceptions with Secondary school mathematics concepts?"
ChatGPT replied "Based on my analysis of web pages and social media exchanges, here are five common misconceptions with Secondary school mathematics concepts:
Misconception 1: Multiplication always results in a larger number. Explanation: Many students assume that multiplying two numbers will always yield a larger value. However, this is not true, as the product can be smaller or larger depending on the values being multiplied. For instance, multiplying a positive number by a fraction less than 1 will result in a smaller value.
Misconception 2: Division always results in a smaller number. Explanation: Similar to the misconception about multiplication, some students believe that dividing two numbers will always yield a smaller value. However, this is not the case, as division can result in a larger or smaller value depending on the numbers involved. For example, dividing a larger number by a smaller number will result in a larger quotient.
Misconception 3: Zero is a positive number. Explanation: Zero is often mistakenly classified as a positive number. However, zero is considered neither positive nor negative; it is its own distinct value. It holds unique properties in mathematics and is crucial in various calculations and number systems.
Misconception 4: Fractions are always smaller than whole numbers. Explanation: Many students perceive fractions as smaller than whole numbers. While it is true that some fractions are less than 1 and appear smaller in value, there are fractions greater than 1 as well. For instance, 3/2 is greater than 1, and 5/4 is greater than 1 as well.
Misconception 5: The concept of a negative number is arbitrary or imaginary. Explanation: Some students struggle with grasping the concept of negative numbers, often considering them arbitrary or imaginary. However, negative numbers have a concrete meaning and significance in various real-life scenarios, such as temperatures below zero or debt. They are an essential part of the number line and mathematical operations."
So the sample used in this survey is hardly representative of all pupils as it is drawing only from those who have used the web to share their thoughts but it is an interesting result nevertheless. Of course it is important to address these misconceptions to help students develop a solid understanding of mathematics.
Finally the answer to last month's puzzle which was:
“This sentence contains _______ letters”
Write a number in words in the blank space in the above sentence that will make the statement true.
Leonard Pomrehn found:
This sentence contains thirty-six letters.
In addition Wil Ransome suggested:
This sentence contains thirty-eight letters.
This sentence contains 27 letters [2 and 7 are not letters].
This sentence contains two score and three letters.
Then Chris Smith who came up with:
This sentence contains thirteen unique letters.
The credit for the puzzle goes to Alex Bellos's Monday Puzzle in The Guardian newspaper
That's all for now,
John
P.S. If a got a pound for every time I failed a maths exam I'd have about £9.30 now.
Do you have any comments? It is always useful to receive feedback on this newsletter and the resources on this website so that they can be made even more useful for those learning Mathematics anywhere in the world. Click here to enter your comments.
