
 |
Exam-Style Questions on FunctionsProblems on Functions adapted from questions set in previous Mathematics exams. |
1. | GCSE Higher |
\(f(x) = \frac{2x}{5} + 7\) and \(g(x) = 10x^2 - 15\) for all values of \(x\).
Find \(fg(x)\).
Give your answer in the form \(ax^2 + b\) where \(a\) and \(b\) are integers.
2. | GCSE Higher |
The function \(f\) is described by the following formula:
$$ f(x) = 3x^2 - 2x^3 $$Calculate the value of \(f(-5) \)
3. | IGCSE Extended |
The table shows some values (rounded to one decimal place) for the function \(y=\frac{2}{x^2}-x, x\neq 0\).
| \(x\) | -3 | -2 | -1 | -0.5 | 0.5 | 1 | 2 | 3 | 4 |
|---|---|---|---|---|---|---|---|---|---|
| \(y\) | 3.2 | 2.5 | 8.5 | 7.5 | 1.0 | -2.8 |
(a) Complete the table of values.
(b) Draw the graph of \(y=\frac{2}{x^2}-x\) for \(-3\le x \le -0.5\) and \(0.5\le x\le 4\).
(c) Use your graph to solve the equation \(\frac{2}{x^2}-x-3=0\)
(d) Use your graph to solve the equation \(\frac{2}{x^2}-x=1-2x\)
(e) By drawing a suitable tangent, find an estimate of the gradient of the curve at the point where x = 1.
(f) Using algebra, show that you can use the graph at \(y=0\) to find \(\sqrt[3]2\)
4. | GCSE Higher |
(a) A function is represented by the following function machine.
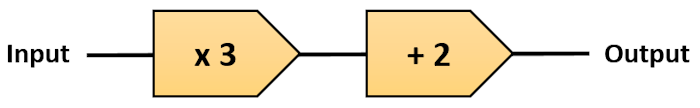
A number is input into the machine and the output is used as a new input.
If the second output is 53 work out the number that was the first input.
(b) A number is input into the machine and the output produced is the same number. Work out what this number could have been.
(c) Another function machine is shown below.
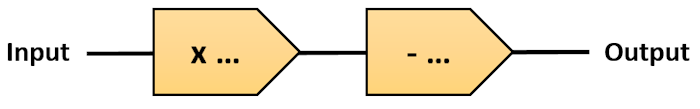
If the Input is 2, the Output is 7.
If the Input is 6, the Output is 27.
Use this information to fill in the two boxes.
5. | IGCSE Extended |
If \(f(x)=5-4x\) and \(g(x)=4^{-x}\) then:
(a) Find \(f(3x)\) in terms of \(x\).
(b) Find \(ff(x)\) in its simplest form.
(c) Work out \(gg(–1)\) give your answer as a fraction.
(d) Find \(f^{–1}(x)\), the inverse of \(f(x)\).
(e) Solve the equation \(gf(x)= 1\).
6. | GCSE Higher |
Here is a function machine that produces two outputs, A and B.
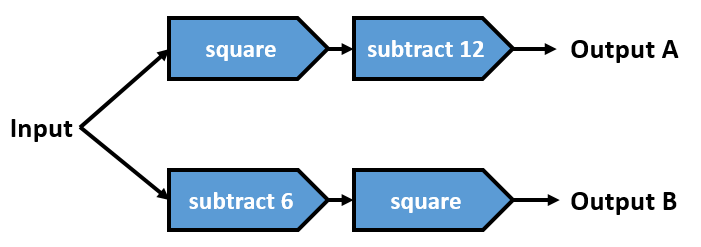
Work out the range of input values for which the output A is less than the output B.
7. | GCSE Higher |
The functions \(f\) and \(g\) are such that:
$$ f(x) = 4x + 3 $$ $$ g(x) = x^2 - 3 $$(a) Find \(f^{-1}(x)\)
(b) Given that \(gf(x) = 3fg(x)\), show that:
$$ 4x^2 + 24x + 33 = 0 $$8. | IB Standard |
Consider the graph of \(f(x)=a\sin(b(x+c))+12\), for \(0\le x\le 24\).
The graph has a maximum at (8, 22) and the next local minimum at (18, 2).
(a) Find the value of \(a\).
(b) Find the value of \(b\).
(c) Find the value of \(c\).
(d) Solve \(f(x)=5\).
9. | IB Analysis and Approaches |
Part of the function of \(y=f(x)\) is shown in the following diagram.
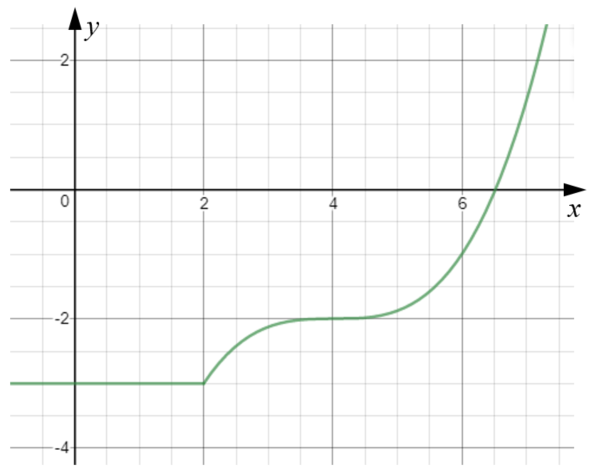
(a) Write down the value of \(f(1)\).
(b) Write down the value of \(ff(7)\).
(c) Sketch the graph of \(g(x)\) if \(g(x) = -f(x)-1\) on the same set of axes above.
10. | IB Standard |
Let \(f (x)=a(x-b)^2+c\). The vertex of the graph of \(f\) is at (4, -3) and the graph passes through (3, 2).
(a) Find the value of \(c\).
(b) Find the value of \(b\).
(c) Find the value of \(a\).
11. | IB Analysis and Approaches |
The functions \(f\) and \(g\) are defined for \(x \in \mathbb{R} \) by
\(f(x) = ax - b\), where \(a, b \in \mathbb{Z} \)
\(g(x) = 2x^2 - x + 5\)
Find the values of \(a\) and \(b\) such that \( (g \circ f)(x) = 18x^2-51x+41\)
12. | IB Applications and Interpretation |
The circumference of a given circle \(C\) can be represented by the function \(C(A) =2\sqrt{A \pi}, A \ge 0 \) where \(A\) is the area of the circle. The graph of the function \(C\) is shown for \(0 \le A \le 16\).
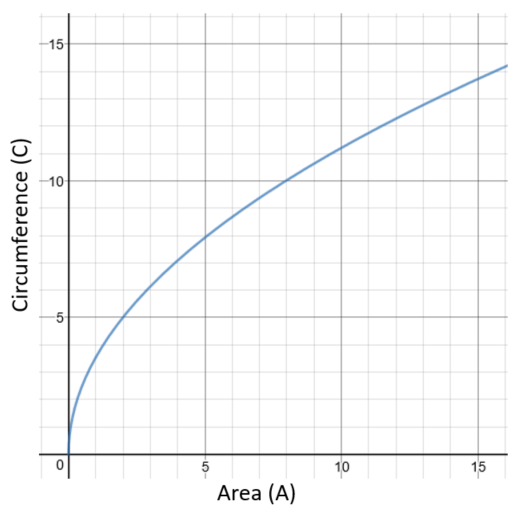
(a) Use the graph to find the value of \(C(8)\) to the nearest whole number.
(b) The range of \(C(A)\) is \(0 \le C(A) \le n\). Write down the value of \(n\).
(c) On the axes above, draw the graph of the inverse function, \(C^{-1}\).
(d) In the context of the question, explain the meaning of \( C^{-1}(12)\).
13. | IB Standard |
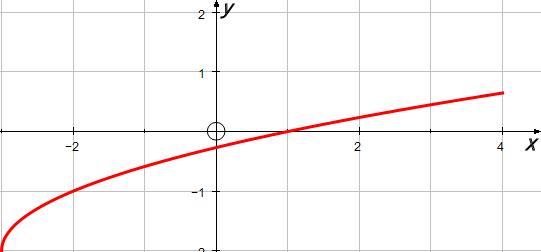
The diagram shows the graph of \(y=f(x)\), for \(-3\le x \le 4\).
The graph passes through the point (4,0.65).
(a) Find the value of \(f(-2)\);
(b) Find the value of \(f^{-1}(0)\);
(c) Find the domain of \(f^{-1}\).
(d) Sketch the graph of \(f^{-1}\).
14. | IB Applications and Interpretation |
The circumference of a given circle \(C\) can be represented by the function \(C(A) = 2 \sqrt{A \pi}\) , \(A \ge 0 \) , where \(A\) is the area of the circle. The graph of the function \(C\) is shown for \(0 \le A \le 10\).
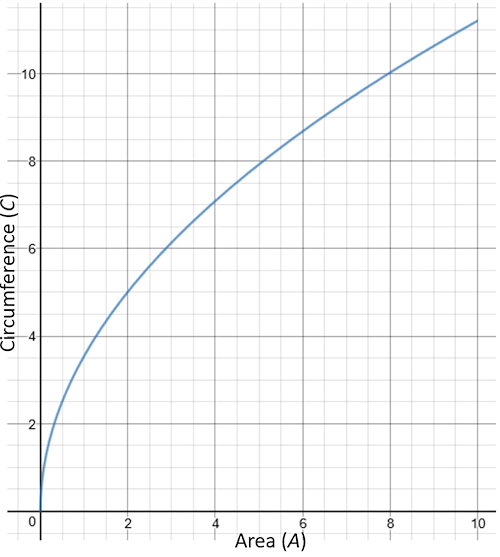
(a) Write down the value of \(C(5)\).
The range of \(C(A)\) is \(0 \le C(A) \le k\).
(b) Find the value of \(k\).
(c) On the axes above, draw the graph of the inverse function, \( C^{-1}\).
(d) In the context of the question, explain the meaning of \( C^{-1}(10) \approx 7.96\).
15. | IB Standard |
The diagram shows part of the graph of \(y=a\sin{(bx)}+c\) with a minimum at \((-2.5,-2)\) and a maximum at \((2.5,4)\).
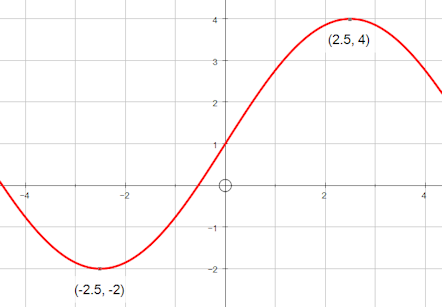
(a) Find \(a\).
(b) Find \(b\).
(c) Find \(c\).
16. | IB Standard |
The Big Wheel at Fantasy Fun Fayre rotates clockwise at a constant speed completing 15 rotations every hour. The wheel has a diameter of 90 metres and the bottom of the wheel is 6 metres above the ground.
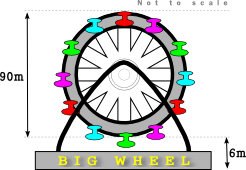
A cabin starts at the bottom of the wheel with the top of the cabin 6m above the ground.
(a) Find the greatest height of the top of the cabin reaches as the wheel rotates.
After \(t\) minutes, the height \(h(t)\) metres above the ground of the top of a cabin is given by the function \(h(t)=51-a\cos bt\).
(b) Find the period of \(h(t)\)
(c) Find the value of \(b\).
(d) Find the value of \(a\).
(e) Sketch the graph of \(h(t)\) , for \(0\le t\le 5\).
(f) In one rotation of the wheel, find the probability that a randomly selected seat is at least 70 metres above the ground. Give your answer to two decimal places.
17. | IB Standard |
The population of sheep on a ranch is modelled by the function \(P(t)= 65 \sin(0.4t-32)+450\), where t is in months, and \(t=1\) corresponds to 1st January 2015.
(a) Find the number of sheep on the ranch on 1st July 2015.
(b) Find the rate of change of the sheep population on 1st July 2015.
(c) Explain your answer to part (b) with reference to the sheep population size on 1st July2015.
18. | IB Standard |
Let \(f(x)=5x^2-20x+k\). The equation \(f(x)=0\) has two equal roots.
(a) Write down the value of the discriminant.
(b) Hence, show that \(k=20\).
The graph of \(f\) has its vertex on the x-axis.
(c) Write down the solution of \(f(x)=0\).
(d) Find the coordinates of the vertex of the graph of \(f\).
The function can be written in the form \(f(x)=a(x-h)^2+j\).
(e) Find the value of \(a\).
(f) Find the value of \(h\).
(g) Find the value of \(j\).
(h) The graph of a function \(g\) is obtained from the graph of \(f\) by a reflection in the x-axis, followed by a translation by the vector \(\begin{pmatrix} 0 \\ 3 \\ \end{pmatrix} \). Find \(g\), giving your answer in the form \(g(x)=Ax^2+Bx+C\).
19. | IB Analysis and Approaches |
The functions f and g are defined as follows:
$$f(x) = \frac{x+4}{5}$$ $$g(x) = 10x - 3$$(a) Find \( (g \circ f)(x) \)
(b) Given that \( (g \circ f)^{-1} (a) = 5 \) , find the value of \(a\).
20. | IB Analysis and Approaches |
The functions \( f \) and \( g \) are defined for \( x \in \mathbb{R} \) by \( f(x) = 3 + 5x - 2x^2 \) and \( g(x) = x + k \), where \( k \in \mathbb{R} \).
(a) Find the range of \( f \).
(b) Given that \( (f \circ g)(x) \) has two distinct roots that sum to zero, determine the value of \( k \).
21. | IB Standard |
The diagram shows part of the graph of \(f(x)=Ae^{kx}+2\).
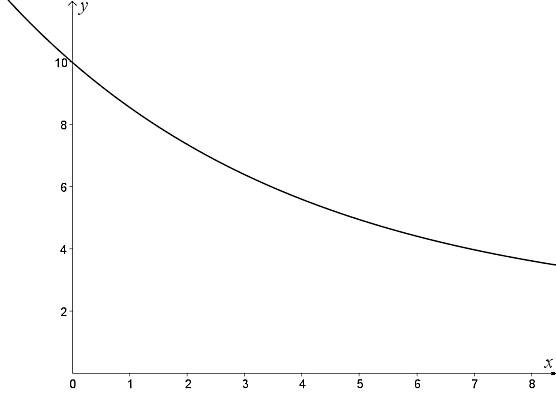
The y-intercept is at \((0,10)\).
(a) Show that \(A=8\).
(b) Given that \(f(8)=3.62\) (correct to 3 significant figures), find the value of \(k\).
(c) (i) Using your value of \(k\), find \(f'(x)\).
(ii) Hence, explain why \(f\) is a decreasing function.
(iii) Find the equation of the horizontal asymptote of the graph \(f\).
Let \(g(x)=-x^2+7x+5\)
(d) Find the area enclosed by the graphs of \(f\) and \(g\).
22. | IB Analysis and Approaches |
The Fun Wheel at the Meller Theme Park rotates at a constant speed.

The height, \(h\) metres, of the point initially at the top of the circumference of the wheel after \(t\) minutes is given by:
$$ h(t) = a \cos(bt) + c $$(a) Find the values of \(a, b \text{ and } c \text{ where } a,b,c \in \mathbb{R} \).
(b) Draw a sketch of the function \(h(t)\) for \(0 < t < 4 \).
23. | IB Standard |
A Big Wheel at an amusement park has a diameter of length 70 metres which rotates at a constant speed. The bottom of the wheel is h metres above the ground. A seat starts at the bottom of the wheel.The wheel completes one revolution in 4 minutes. [The diagram is not to scale]
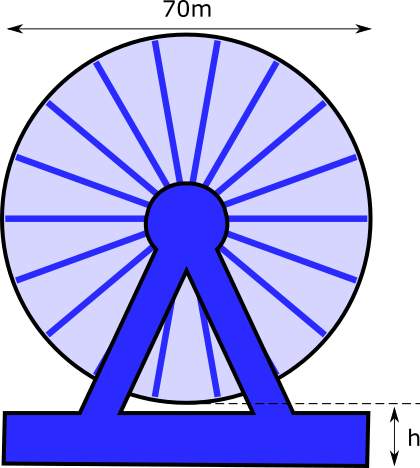
(a) After 2 minutes, the seat is 76m above the ground. Find h.
(b) After t minutes, the height of the seat above ground is given by \(f(t) =41+ k \cos{ \frac{\pi t}{2}} \) for \(0 \le t \le 16 \).
Find the value of k.
(c) Find when the seat is 40 m above the ground for the third time.
24. | IB Standard |
Part of the graph of \(f(x) = {\log _b}(x + 4)\) for \(x > - 4\) is shown below.
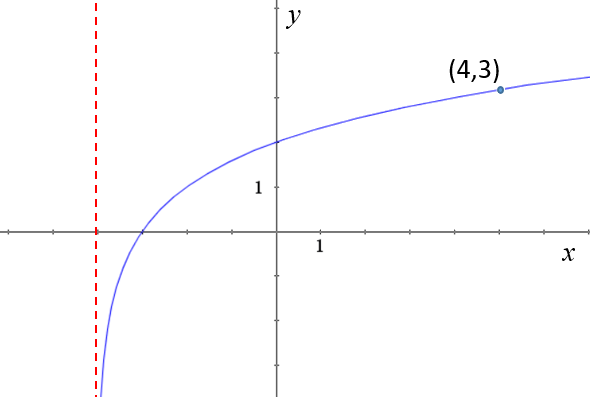
The graph passes through A(4, 3) , has an x-intercept at (-3, 0) and has an asymptote at \(x = - 4\).
(a) Find the value of \(b\).The graph of \(f(x)\) is reflected in the line \(y = x\) to give the graph of \(g(x)\).
(b) Write down the y-intercept of the graph of \(g(x)\).
(c) Sketch the graph of \(g(x)\), noting clearly any asymptotes and the image of A.
(d) Find \(g(x)\) in terms of \(x\).
25. | A-Level |
The functions \(f\) and \(g\) are defined as:
$$ f(x) = \sqrt{3x+4} \text{ for } x \ge -1 $$ $$ g(x) = x^2 - 3x \text{ for all real values of }x $$(a) Find \( f^{-1}(x) \)
(b) State the domain of \( f^{-1}\).
(c) Find the range of \(g\).
(d) Find \(gf(x)\).
(e) Solve the equation \(gf(x) = 10\).
26. | IB Standard |
Let \(f(x)=\sin ( \frac {\pi}{4}x) + \cos ( \frac {\pi}{4}x) \), for \(-4\le x \le 4\)
(a) Sketch the graph of \(f\).
(b) Find the values of \(x\) where the function is decreasing.
(c) The function \(f\) can also be written in the form \(f(x)=a\sin ( \frac {\pi}{4}(x+c))\) where \(a\in \mathbb R\) and \(0 \le c \le 2\). Find the value of \(a\) and \(c\).
27. | IB Analysis and Approaches |
Consider the function \(f(x) = k^x \) where \(x, k \in \mathbb{R}\) and \( x \gt 0, k > 1\).
The graph of \(f\) passes through the point \( (\frac{3}{2},27) \).
(a) Find the value of \(k\).
(b) Write down an expression for \( f^{-1}(x) \).
(c) Find the value of \( f^{-1}( \frac{1}{3}) \).
28. | A-Level |
The function \(f\) is defined as \(f(x) = 12x^3 - 5x^2 -11x + 6 \).
(a) Use the Factor Theorem to show that \( (4x-3) \) is a factor of \(f(x)\)
(b) Express \(f(x)\) as a product of linear factors.
(d) Hence solve the equation \( g(\theta ) = 0 \), giving your answers, in radians, in the interval \(0 \le \theta \le 2 \pi \).
29. | IB Analysis and Approaches |
Consider a function \(f\), such that \(f(x)=7.2\sin(\frac{\pi}{6}x + 2) + b\) where \( 0\le x \le 12\)
(a) Find the period of \(f\).
The function \(f\) has a local maximum at the point (11.18,10.3) , and a local minimum at (5.18.-4.1).
(b) Find the value of b.
(c) Hence, find the value of \(f(7)\).
A second function \(g\) is given by \(g(x)=a\sin(\frac{2\pi}{7}(x -4)) + c\) where \(0 \le x \le 10\)
The function \(g\) passes through the points (2.25,-3) and (5.75,7).
(d) Find the value of \(a\) and the value of \(c\).
(e) Find the value of x for which the functions have the greatest difference.
30. | IB Analysis and Approaches |
A function \( g \) is defined by \( g(x) = \frac{3x - 10}{x^2 - 9} \), where \( x \in \mathbb{R} \), \( x \neq \pm3 \).
(a) Determine the range of \( g \).
A function \( h \) is defined by \( h(x) = g(|x|)\sin(t) \), where \( x \in \mathbb{R} \), \( x \neq \pm3 \) and \( t \) is a constant where \( -\pi < t < 0 \).
(b) Find the set of values of \( x \) such that \( h(x) \geq 0 \).
31. | IB Analysis and Approaches |
A carriage attached to a tall vertical pole in an amusement park ride whisks customers up and down. The height, \(H\) metres, of the base of the carriage above the ground can be modelled by the function \(H(t) = a\cos(0.4t) + b\), for \(a, b \in \mathbb{R}\) and \(t\) is the time in seconds after the ride starts.
(a) Find the period of the function.

The ride begins when its base is at a minimum height of 1 metre above the ground, and it reaches a maximum height of 31 metres above the ground.
(b) Find the values of a and b.
(c) Find the number of times that the carriage reaches its maximum height in the first minute of its motion.
(d) Find the first time that the base of the carriage reaches a height of 15 metres.
A camera is set to take a picture of the ride at a random time during the first 15 seconds of its motion.
(e) Find the probability that the height of the base of the carriage is greater than 10 metres at the time the picture is taken.
32. | IB Analysis and Approaches |
A function \( f \) is defined by \( f(x) = \arccos\left(\frac{x^2}{1-x^4}\right) \), \( x \in \mathbb{R} \), \( 0.785 \lt |x| \lt 5\).
(a) Show that \( f \) is an even function.
(b) Find the equations of the horizontal asymptote of \( y = f(x) \).
(c) Find \( f'(x) \) for \( x \in \mathbb{R} \), \( x \neq \pm1 \).
(d) Determine whether \( f \) is increasing or decreasing for \( x > 1 \).
(e) Find an expression for \( f^{-1}(x) \), for \( x \in \mathbb{R} \), \( x \neq \pm1\)
33. | A-Level |
The height above the ground, H metres, of a passenger on a Ferris wheel t minutes after the wheel starts turning, is modelled by the following equation:
$$H = k – 8\cos (60t)° + 5\sin (60t)°$$where k is a constant.
(a) Express \(H\) in the form \(H = k - R \cos(60t + a)° \) where \(R\) and \(a\) are constants to be found (\( 0° \lt a \lt 90° \)).
(b) Given that the initial height of the passenger above the ground is 2 metres, find a complete equation for the model.
(c) Hence find the maximum height of the passenger above the ground.
(d) Find the time taken for the passenger to reach the maximum height on the fifth cycle. (Solutions based entirely on graphical or numerical methods are not acceptable.)
(e) It is decided that, to increase profits, the speed of the wheel is to be increased. How would you adapt the equation of the model to reflect this increase in speed?
34. | IB Analysis and Approaches |
The height of water in metres above Chart Datum* in the Port of Bristol on a day in January is modelled by the function:
$$H(x) = a \sin(b(x-c)) +d$$where \(x\) is the number of hours after midnight GMT, and \(a,b,c, \text{ and } d \) are constants greater than zero.
The following graph shows the height of the water for 19 hours starting at midnight.
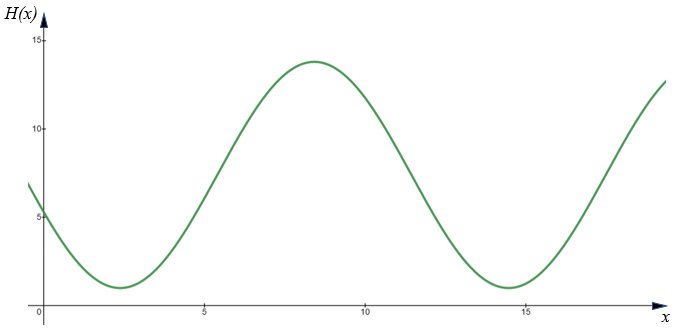
The first low tide occurs at 02:23 and next low tide occurs at 14:28.
Throughout the day the height of the water fluctuates between 1m and 13.8m.
(a) Show that \(a = 6.4\).
(b) Find the value of \(b\) to two decimal places.
(c) Find the value of \(d\).
(d) Find the smallest possible value of \(c\) to one decimal place.
(e) Using the values of \(a,b,c, \text{ and } d \) found above, find the height of the water at 10:00.
(f) Determine the number of hours, during this January day, for which the tide is higher than 10 metres above Chart Datum.
* Chart Datum is a reference datum or level from which heights and depths on a nautical chart are measured. Chart Datum is determined by the lowest astronomical tide, which is the level of the sea at its lowest possible level. It is also the reference level used to measure the depth of water in a harbour or other body of water.
35. | IB Analysis and Approaches |
Consider the function \(f(x) = \dfrac{x^3+2x}{5}, \; x \in \mathbb{R}\).
(a) Show that \(f\) is an odd function.
The function \(g\) is given by:
$$ g(x) = \dfrac{3x-3}{x^2+x-2} \quad \text{ where } x \in \mathbb{R}, x \neq 1, \; x \neq -2.$$ (b) Solve the inequality \( f(x) \lt g(x) \).If you would like space on the right of the question to write out the solution try this Thinning Feature. It will collapse the text into the left half of your screen but large diagrams will remain unchanged.
The exam-style questions appearing on this site are based on those set in previous examinations (or sample assessment papers for future examinations) by the major examination boards. The wording, diagrams and figures used in these questions have been changed from the originals so that students can have fresh, relevant problem solving practice even if they have previously worked through the related exam paper.
The solutions to the questions on this website are only available to those who have a Transum Subscription.
Exam-Style Questions Main Page
To search the entire Transum website use the search box in the grey area below.
Do you have any comments about these exam-style questions? It is always useful to receive feedback and helps make this free resource even more useful for those learning Mathematics anywhere in the world. Click here to enter your comments.