Here are some specific activities, investigations or visual aids we have picked out. Click anywhere in the grey area to access the resource.
 Differentiation Video A reminder of how to differentiate different types of functions and how to find the equations of tangents and normals.
Differentiation Video A reminder of how to differentiate different types of functions and how to find the equations of tangents and normals.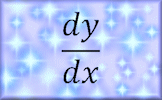 Differentiation Practise the technique of differentiating polynomials with this self marking exercise.
Differentiation Practise the technique of differentiating polynomials with this self marking exercise.
Click on a topic below for suggested lesson Starters, resources and activities from Transum.
Furthermore
The concept of a limit in mathematics describes the value that a function approaches as its input approaches a certain value. It's a foundational idea in calculus, allowing us to understand how functions behave near particular points, even if they're undefined at those points. The derivative of a function, on the other hand, represents the rate at which the function is changing. When interpreted geometrically, the derivative at a point gives the gradient (or slope) of the tangent to the curve of the function at that point. In real-world applications, the derivative often represents a rate of change, such as how an object's velocity changes over time.
Key Formulae:
$$ \text{Limit:} \quad \lim_{{x \to a}} f(x) = L $$
$$ \text{Derivative:} \quad f'(x) = \lim_{{h \to 0}} \frac{f(x+h) - f(x)}{h} $$
Example:
Consider the function \( f(x) = x^2 \). Let's find the derivative of this function at the point \( x = 2 \).
Using the definition of the derivative:
$$ f'(2) = \lim_{{h \to 0}} \frac{(2+h)^2 - 2^2}{h} \\ f'(2) = \lim_{{h \to 0}} \frac{4h + h^2}{h} \\ f'(2) = \lim_{{h \to 0}} (4 + h) \\ f'(2) = 4 $$
This means that the gradient of the tangent to the curve \( y = x^2 \) at the point \( x = 2 \) is 4.
If you use a TI-Nspire GDC there are instructions useful for this topic.
This video on differentiation is from Revision Village and is aimed at students taking the IB Maths Standard level course.
How do you teach this topic? Do you have any tips or suggestions for other teachers? It is always useful to receive feedback and helps make these free resources even more useful for Maths teachers anywhere in the world. Click here to enter your comments.

